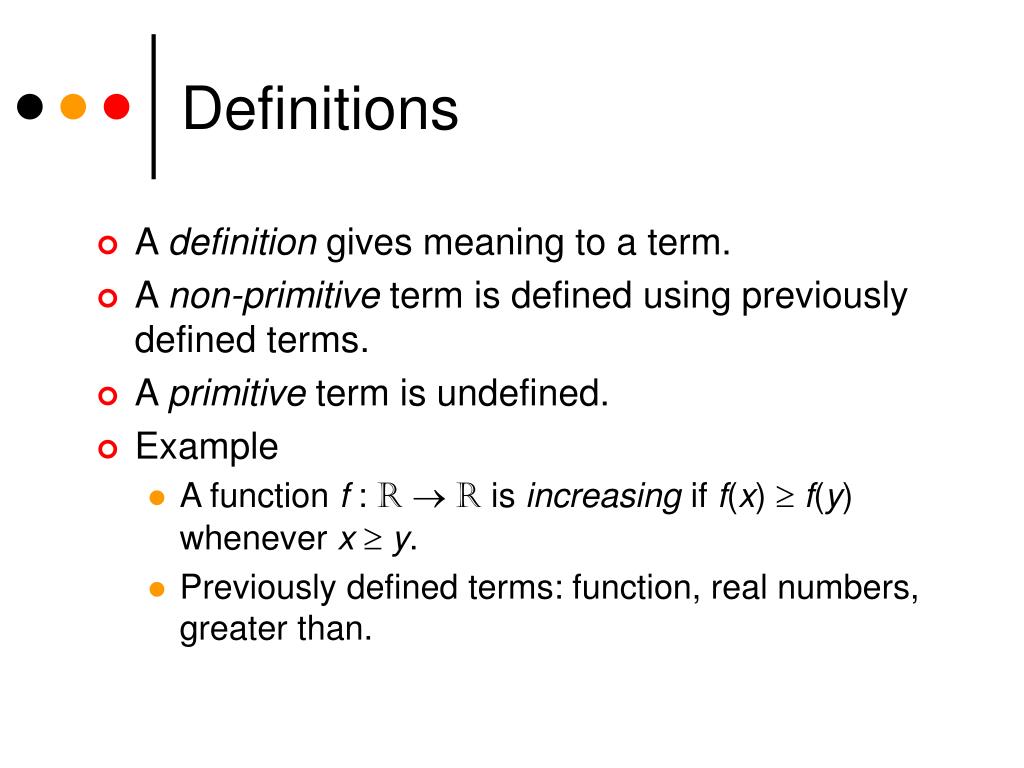
Proof By Counterexample Example . The existence of even one such counterexample means that the. 1 x +1 = x +1 x. For example, here is an algebraic identity for real numbers: It is merely a way of showing that a given statement cannot possibly be. A proof by counterexample is not technically a proof. Showing that a mathematical statement is true requires a formal proof. Prove or disprove the following statements using the method of direct proof or counterexample. The difference of any two odd integers is odd. It is true for all x 6= 0. However, showing that a mathematical statement is false only requires. In exercise 6.12.8, you are asked to prove the following statement by proving the contrapositive. But to prove that such a claim is false, it suffices to find a single counterexample. Since an algebraic identity is a statement about.
from www.slideserve.com
Since an algebraic identity is a statement about. 1 x +1 = x +1 x. A proof by counterexample is not technically a proof. Showing that a mathematical statement is true requires a formal proof. It is true for all x 6= 0. It is merely a way of showing that a given statement cannot possibly be. For example, here is an algebraic identity for real numbers: But to prove that such a claim is false, it suffices to find a single counterexample. The existence of even one such counterexample means that the. The difference of any two odd integers is odd.
PPT Direct Proof and Counterexample I PowerPoint Presentation, free
Proof By Counterexample Example For example, here is an algebraic identity for real numbers: It is merely a way of showing that a given statement cannot possibly be. 1 x +1 = x +1 x. For example, here is an algebraic identity for real numbers: Since an algebraic identity is a statement about. The existence of even one such counterexample means that the. But to prove that such a claim is false, it suffices to find a single counterexample. Showing that a mathematical statement is true requires a formal proof. In exercise 6.12.8, you are asked to prove the following statement by proving the contrapositive. However, showing that a mathematical statement is false only requires. Prove or disprove the following statements using the method of direct proof or counterexample. It is true for all x 6= 0. A proof by counterexample is not technically a proof. The difference of any two odd integers is odd.
From www.youtube.com
Proof (P2 Proof by Exhaustion and Counterexample) Algebraic Methods Proof By Counterexample Example The existence of even one such counterexample means that the. 1 x +1 = x +1 x. For example, here is an algebraic identity for real numbers: Since an algebraic identity is a statement about. The difference of any two odd integers is odd. But to prove that such a claim is false, it suffices to find a single counterexample.. Proof By Counterexample Example.
From slideplayer.com
Direct Proof and Counterexample I ppt download Proof By Counterexample Example For example, here is an algebraic identity for real numbers: But to prove that such a claim is false, it suffices to find a single counterexample. A proof by counterexample is not technically a proof. 1 x +1 = x +1 x. It is merely a way of showing that a given statement cannot possibly be. Since an algebraic identity. Proof By Counterexample Example.
From www.youtube.com
Counterexample YouTube Proof By Counterexample Example It is true for all x 6= 0. For example, here is an algebraic identity for real numbers: Prove or disprove the following statements using the method of direct proof or counterexample. But to prove that such a claim is false, it suffices to find a single counterexample. The existence of even one such counterexample means that the. A proof. Proof By Counterexample Example.
From www.slideshare.net
Dec.10 Counterexamples Proof By Counterexample Example The difference of any two odd integers is odd. A proof by counterexample is not technically a proof. However, showing that a mathematical statement is false only requires. 1 x +1 = x +1 x. Since an algebraic identity is a statement about. In exercise 6.12.8, you are asked to prove the following statement by proving the contrapositive. But to. Proof By Counterexample Example.
From www.slideserve.com
PPT Direct Proof and Counterexample I PowerPoint Presentation, free Proof By Counterexample Example In exercise 6.12.8, you are asked to prove the following statement by proving the contrapositive. Since an algebraic identity is a statement about. It is true for all x 6= 0. Showing that a mathematical statement is true requires a formal proof. However, showing that a mathematical statement is false only requires. Prove or disprove the following statements using the. Proof By Counterexample Example.
From www.slideserve.com
PPT Methods of Proof PowerPoint Presentation, free download ID226798 Proof By Counterexample Example It is merely a way of showing that a given statement cannot possibly be. 1 x +1 = x +1 x. The existence of even one such counterexample means that the. Prove or disprove the following statements using the method of direct proof or counterexample. It is true for all x 6= 0. But to prove that such a claim. Proof By Counterexample Example.
From www.slideserve.com
PPT Introduction to Proofs PowerPoint Presentation, free download Proof By Counterexample Example In exercise 6.12.8, you are asked to prove the following statement by proving the contrapositive. However, showing that a mathematical statement is false only requires. But to prove that such a claim is false, it suffices to find a single counterexample. Prove or disprove the following statements using the method of direct proof or counterexample. A proof by counterexample is. Proof By Counterexample Example.
From www.slideserve.com
PPT Direct Proof and Counterexample II PowerPoint Presentation, free Proof By Counterexample Example However, showing that a mathematical statement is false only requires. 1 x +1 = x +1 x. Prove or disprove the following statements using the method of direct proof or counterexample. Showing that a mathematical statement is true requires a formal proof. The existence of even one such counterexample means that the. A proof by counterexample is not technically a. Proof By Counterexample Example.
From www.slideserve.com
PPT 4.1 Proofs and Counterexamples PowerPoint Presentation, free Proof By Counterexample Example Prove or disprove the following statements using the method of direct proof or counterexample. The difference of any two odd integers is odd. Since an algebraic identity is a statement about. In exercise 6.12.8, you are asked to prove the following statement by proving the contrapositive. Showing that a mathematical statement is true requires a formal proof. It is true. Proof By Counterexample Example.
From www.slideserve.com
PPT Proof Techniques PowerPoint Presentation, free download ID1968391 Proof By Counterexample Example The existence of even one such counterexample means that the. 1 x +1 = x +1 x. Prove or disprove the following statements using the method of direct proof or counterexample. However, showing that a mathematical statement is false only requires. For example, here is an algebraic identity for real numbers: A proof by counterexample is not technically a proof.. Proof By Counterexample Example.
From studywell.com
Mathematical Proof Examples ExamStyle Questions StudyWell Proof By Counterexample Example For example, here is an algebraic identity for real numbers: It is merely a way of showing that a given statement cannot possibly be. In exercise 6.12.8, you are asked to prove the following statement by proving the contrapositive. A proof by counterexample is not technically a proof. Showing that a mathematical statement is true requires a formal proof. The. Proof By Counterexample Example.
From www.slideserve.com
PPT Direct Proof and Counterexample I PowerPoint Presentation, free Proof By Counterexample Example Showing that a mathematical statement is true requires a formal proof. In exercise 6.12.8, you are asked to prove the following statement by proving the contrapositive. Prove or disprove the following statements using the method of direct proof or counterexample. Since an algebraic identity is a statement about. The existence of even one such counterexample means that the. For example,. Proof By Counterexample Example.
From www.cuemath.com
Counterexample Cuemath Proof By Counterexample Example The existence of even one such counterexample means that the. However, showing that a mathematical statement is false only requires. Since an algebraic identity is a statement about. For example, here is an algebraic identity for real numbers: Showing that a mathematical statement is true requires a formal proof. But to prove that such a claim is false, it suffices. Proof By Counterexample Example.
From www.slideserve.com
PPT Section One PowerPoint Presentation, free download ID1347096 Proof By Counterexample Example However, showing that a mathematical statement is false only requires. It is true for all x 6= 0. 1 x +1 = x +1 x. Prove or disprove the following statements using the method of direct proof or counterexample. The existence of even one such counterexample means that the. A proof by counterexample is not technically a proof. For example,. Proof By Counterexample Example.
From www.slideserve.com
PPT Direct Proof and Counterexample III PowerPoint Presentation, free Proof By Counterexample Example A proof by counterexample is not technically a proof. Since an algebraic identity is a statement about. It is merely a way of showing that a given statement cannot possibly be. 1 x +1 = x +1 x. But to prove that such a claim is false, it suffices to find a single counterexample. However, showing that a mathematical statement. Proof By Counterexample Example.
From www.slideserve.com
PPT Direct Proof and Counterexample I PowerPoint Presentation, free Proof By Counterexample Example It is true for all x 6= 0. In exercise 6.12.8, you are asked to prove the following statement by proving the contrapositive. 1 x +1 = x +1 x. Prove or disprove the following statements using the method of direct proof or counterexample. For example, here is an algebraic identity for real numbers: But to prove that such a. Proof By Counterexample Example.
From www.youtube.com
P2 new Practice Paper A IAL Q2 Disproof by Counter Example and Proof by Proof By Counterexample Example The existence of even one such counterexample means that the. Prove or disprove the following statements using the method of direct proof or counterexample. 1 x +1 = x +1 x. Showing that a mathematical statement is true requires a formal proof. It is merely a way of showing that a given statement cannot possibly be. For example, here is. Proof By Counterexample Example.
From www.slideserve.com
PPT Direct Proof and Counterexample I PowerPoint Presentation, free Proof By Counterexample Example A proof by counterexample is not technically a proof. It is true for all x 6= 0. However, showing that a mathematical statement is false only requires. It is merely a way of showing that a given statement cannot possibly be. Showing that a mathematical statement is true requires a formal proof. The difference of any two odd integers is. Proof By Counterexample Example.